6 x 4 means…
So, while casually browsing Twitter, I noticed this tweet:
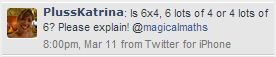
This is something I was actually thinking about a little while ago so I replied with “bearing in mind the answers the same of course. I’d say you start with 6 and then multiply by 4. So 4 lots of 6.”
@PlussKatrina: I understand it’s commutative just want to make sure I model it correctly to the children- drawing 4 groups of 6 yes?
Me: You need a primary specialist. I can’t see it makes any difference really.
@MichaelaG67: I’d say 4 lots of 6 as the x sign is indicating 4 times but I could be wrong too.
@PlussKatrina: just want to make sure I don’t want to create misconceptions for when we move onto division and further through education!
So that seems to all tie up nicely, and then…
@ptompkins7: 6×4 is 6 groups of 4. Helps to record as 6 fours. The unit is “four”. Same for 3 “tenths”, 5″cm”, 7″x” etc
Which makes it safe to say there’s not a clear consensus.
My thinking goes as follows. When looking at 7 + 3, I think of starting with 7 and then physically adding 3. With 12 – 8 I think of having 12 things and removing 8. Similarly, with dividing, 24/4 I start with 24 and the act is ‘dividing it into 4 equal parts’. So, in general, you start with the first number and ‘do’ with the second.
I’d argue that 6×4 suggests you start with 6 and then ‘do making this four times as much’. For example, that means starting with six things in a box and then getting four of those boxes.
A quick search on Wolfram Alpha gives this illustration:
Which seems pretty inconclusive. It’s hard to tell which way round this is. Is it a row of 6 blocks, stacked up four high or a column of 4 repeated 6 times. Who knows?
My one issue is that 6×4, the way I’ve described suggests “4 lots of 6” as a way of saying it and that requires saying the numbers in the wrong order. I don’t like that.
So, in conclusion, following what I said about adding, subtracting and dividing, I think my way of thinking is sensible. Considering implications for higher level maths, if we are talking about 5 lots of k or k lots of 5, we write 5k either way so I don’t think it’s really an issue.
Any strong opinions out there?

There is no correct answer for 6×4 being 6 times four, 6 multiplied by 4 or 6 lots of 4 or 4 lots of 6. These arrays (images like the one shown) are great for showing the commutative element of multiplication. Also link really well with division so using the same image and split it into 4 groups or 6 groups. You can have some very rich conversations with pupils around this basic image! I have used real life examples as well such as eggs in a tray or milk cartoons lined up in a cage.
Surely there’s a ‘correct’ answer 😉
I do worry more about potential misconceptions over division tbh.
I think the commutative nature of multiplication makes it irrelevant, and I think the feelings either way will probably stem from how someone learns their times tables. When I was a child we learned 1X2=2, 2X2=4, 3X2=6. However, when my mum was growing up in Scotland she was taught: 2×1=2, 2X2=4, 2×3=6 etc. I would think if you learned them my way, you would be inclined to think of 1×2 as 1 lot of two, 4×6 as 4 lots of six, as the second number is the constant who’s times table you are going up. But if you learned my mum’s was, the first number is the ones who’s times table you’re in so 6×4 would then be four lots of six.
I think the concern over misconceptions comes from the right place, but I really can’t see it being an issue at all.
Thanks cav. I’m inclined to agree.
I think as long as you talk this through with pupils – get them to draw it out and see that 6 lots of 4 and 4 lots of 6 are different things, but which do lead to the same answer – then either way is okay as long as it’s consistent (so then what you conclude above would make a lot of sense).
That way, when you get to division, they are more ready to notice the difference between 24 / 8 and 24 / 3 (not that these two calculations give the same answer, but that they can cause confusion because they are part of the same multiplication fact, and the picture that they make looks similar).
I guess what I’m saying is that: if they don’t think it matters which way round things happen in multiplication, they can get confused when it does matter in division (possibly one of the causes of pupils writing 8 / 24 = 3?). There’s definitely some kind of missing link when (particularly lower set) pupils learn division.
Interesting thoughts Nyima. Is the same true for adding and subtracting? Is it just a matter of experience that for some things, the order makes a difference and for others it doesn’t?
If anything, I think my suggestion would help to reinforce that you start with the first thing and ‘do’ the second thing so to avoid that confusion for division.
Agreed – I suppose you begin with the understanding that one starts with the first number and “does” the operation to it using the second number. I think you have to establish this meaning clearly at first.
But when this is understood, you can start to do what mathematics does best: manipulate the structure of things to discover more efficient methods. e.g at first “24-23” is exactly that: “take 23 away from 24” But as experience is gained, we understand that it is possible to manipulate that expression and simply count on from 23 to 24. Technically, we haven’t answered the original question, but found an equivalent answer through a more efficient method.
On the one had they seems like unimportant differences, but on the other I suspect that they lie at the heart of many misconceptions about maths.
6×4 = 6 lots of 4 = 4+4+4+4+4+4. Similarly, 3x is best thought of as x+x+x, rather than 3+3+3+…+3 (x times). (Additionally, IIRC, when you stray into matrix multiplication, multiplying from the left is used the most.)
Hmm. The 3x argument is pretty compelling.
I agree that it’s more intuitively to think of 3x as x+x+x, but a student understanding the concept should switch easily, when required, to think of it as 3+3+…+3 (x times) and understand that this thinking is applicable only when x is a positive integer.
By the way, do we teach students in Primary school that multiplication is a repetitive addition? We can argue here that the approach would not allow the students to make the difference between the additive and multiplicative thinking. If multiplication is just repetitive addition, why need the proportional thinking?
I see 4×6 more as the 4 enlarged 6 times, which is the same as 6 enlarged 4 times.
Reblogged this on The Echo Chamber.
I think Euclid treats multiplication as area, and does arithmetic via geometry as a concrete model, so the question arises very differently, and the “rectangle theorem” is basic. The Greeks and Romans had number systems which didn’t lend themselves easily to systematic multiplication, so the way we treat this is modern. I learned “One four is four, two fours are eight, three fours are twelve …” – if you teach tables like that then you only have one choice for the primary meaning. One of the reasons multiplication is useful is that both statements are true.
I’m not sure I can add to the excellent discussion above but you asked for my views so here they are.
I’m not a school teacher but I naturally think of 4×6 as 4 lots of 6 and illustrate with 4 rows of six dots. I see this as multiples or repeated addition so it is natural to extend to 4×6.5 but not 6.5×4 until the commutative property has been established (using arrays of dots or areas of rectangles). This discussion has prompted me to consider why I think of it this way round and does it matter. I think my preference comes from the fact that we tend to write scalars on the left, so I’m agreeing with the 3x being x+x+x comment above. Does it matter? Probably not – it’s just a convention. Provided you are consistent then the pupils will quickly reach the stage where they see 4×6.5 and 6.5×4 as one and the same.
As a footnote, I once saw the commutative property explained by two pictures one of which displayed 3 trees each containing 2 birds whilst the other was 2 trees each containing 3 birds. My daughter asked me how this showed that 2×3 was the same as 3×2. I still don’t know the answer to that one!
I think it’s really important for kids to learn very early on that addition and multiplication are commutative, but subtraction and division are not.
You can have a discussion about whether 6×4 is 4+4+4+4+4+4 or 6+6+6+6, but that discussion should end with kids seeing that it’s exactly the same calculation … in just the same way that 3+7 is exactly the same sum as 7+3.
It makes a difference when you’re multiplying ordinals. 2(omega) and (omega)2 are different.
See http://en.wikipedia.org/wiki/Ordinal_arithmetic#Multiplication
This isn’t something that would drive me to claim there was a “right” way to look at 4×6 though.
I see it as “6 fours”, just to confuse things more. “x” being “lots of”. As in, 6×4 is 6 lots of 4, or 6 times 4 things (6 packets of 4 pens) rather than 6 things times 4 (4 packets of 6 pens). But yes, regardless, we still get 24 pens either way!